Towards a modernized geodetic datum for Nepal

Options for developing an accurate terrestrial reference frame following the April 25, 2015 Mw7.8 Gorkha earthquake

Chris Pearson School of Surveying, University of Otago, New Zealand

Niraj Manandhar Survey Department, Nepal

Along with the damage to buildings and infrastructure, the April 25, 2015 Mw7.8 Gorkha earthquake caused quite significant deformation over a large area in central Nepal with displacements of over 2 m recorded in the vicinity of Kathmandu. Correcting for this will require a national deformation mode (NDM) that will have the capacity to correct for the earthquake displacements and ongoing tectonic deformation associated with Nepal’s location on the India/Eurasian plate boundary. The NDM discussed here contains models of the velocity field and co-seismic deformation. The velocity model for Nepal is based on a compilation of published velocity measurements used to study the boundary between the Indian plate to the south and the overriding Eurasian plate to the north. The coseismic deformation associated with the Gorkha earthquake and its 12th May Mw7.3 aftershock was modeled using published dislocation models. By combing the velocity and co-seismic models we have developed an NDM that can correct coordinate for both the effect of the earthquakes and continuous deformation associated with Indian / Eurasian plate boundary. Preliminary tests of the model demonstrate that applying the NDM makes a significant improvement when adjusting survey data sets that were acquired both before and after the earthquakes.

Introduction

The current Nepal-Everest datum is a classical datum developed in 1984 by the Military Survey branch of the Royal (UK) Engineers in collaboration with the Nepal Survey Department, (Spence 1987). However, Nepal is located at the conjoint of two converging plates: the Indian plate to the south and the overriding Eurasian plate to the north. Due to the regular convergence of these plates the existing passive geodetic control network has become distorted with time. This combined with the effect of the April 25, 2015 Mw7.8 Gorkha earthquake, which caused significant deformation over a large area centered on Kathmandu means that the integrity of the current Nepal-Everest datum cannot be assured. In this paper we consider options for a modernized geodetic datum for Nepal that will have the capacity to correct for the earthquake displacements and ongoing tectonic deformation associated with Nepal’s location on the India/Asia plate boundary.

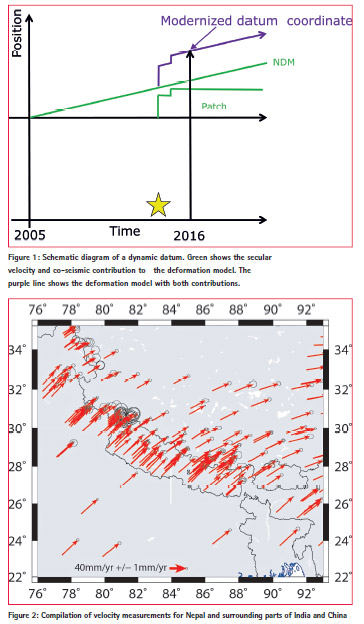
Semi-dynamic vs conventional datums

Because of the effect of plate tectonic motions, the actual position of points on the earth change continuously. However nearly all users find it difficult to deal with continuous coordinate change. There are two quite different ways that geodetic datums can deal with tectonic motion. Modern semi-dynamic datums are based on a version of the International Terrestrial Reference Frame. Stable coordinates are produced by projecting each coordinate to its position at a common date called the reference epoch. In order to make this technique work we need a model of how the earth is moving due to plate tectonics. In stable areas, the effect of earthquakes will be small and the motion of the points will follow the motion of the tectonic plates. In areas that are located on the boundaries of tectonic plates, the motion is more complicated because the points are deforming or moving relative to each other in complex ways. In this case a mathematical model, usually called a National Deformation Model (NDM), is used to calculate the trajectory of points. This usually includes a way of estimating the constant or secular velocity of each point and a way of calculating the effect of any earthquakes that may have occurred between the time that the coordinates were measured (epoch of observation) and the reference epoch. The effect of earthquakes is an instantaneous offset while the effect of the velocity increases linearly with time. This process is illustrated in Figure 1, which shows the trajectory of a point effected by a constant velocity and two earthquake shifts.

In contrast, older classical datums, which were usually established before the reality of plate tectonics was widely accepted, establish fix coordinates for a network of control points with no mechanism to correct for tectonic motion. As a result the marks will drift off their true position. However, the relative position of points will not change significantly with time in stable areas such as the interiors of plates since the entire region is moving rigidly. However, for regions on plate boundaries such as Nepal, ongoing deformation means that the relative position of points will change with time due to an inhomogeneous velocity field and earthquakes. Thus the datum will become distorted as the bearings and distances between marks calculated from their coordinates become increasingly different from what we would measure on the ground.

Semi-dynamic datum for Nepal

Nepal is located at the conjoint of two converging plates: the Indian plate to the south and the overriding Eurasian plate to the north. A significant amount of the convergent component of plate motion is accommodated within Nepal resulting in the crustal velocities changing from a northeast trend in Northern India to an east-northeast trend in southern Tibet. Due to the regular convergence of these plates Nepal has been subjected to a series of great earthquakes including the 25th April 2015 Mw7.8 Gorkha earthquake. Thus, correcting coordinates to a reference epoch requires that a semidynamic datum for Nepal incorporates a National Deformation model . In Nepal’s case the NDM must contain a model of the variation of the long term (or secular) crustal velocity across the country and the co-seismic deformation associated with the April 25, 2015 Mw7.8 Gorkha earthquake and the 12th May Mw7.3 aftershock. Both the velocity model and the co-seismic deformation models are grid files so that the estimates of the velocity or co-seismic shifts can be determined using a process of linear interpolation (Stanaway et al. 2012).

Our model of the velocity field for Nepal was developed by combining published velocities for Nepal and adjacent parts of China and India from four geodetic studies in the Nepal region (see Pearson et al 2016 for a detailed discussion). The velocities are shown in Figure 2.

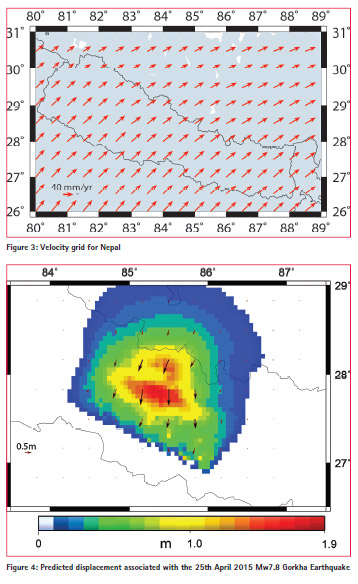
Using these velocities we developed a grid file that covers the region from 80°E to 89°E and 26°N to 31°N (Figure 3). While Figure 3 shows velocity vectors on a half degree spacing the actual gridded velocities have a spacing of 20 points/degree (0.05°).

An NDM also must include patches or gird files that can be used to predict the earthquake deformation at any point. We are currently working on developing patches for the co-seismic part of the 25th April 2015 Gorkha Earthquake and the 12th May Mw7.3 aftershock using published dislocation models (Galetzka et al., 2015). Figure 4 shows the co-seismic slip from the 25th April 2015 Gorkha Earthquake. Note that the Kathmandu Valley has moved by up to 1.9 m.

The deformation model would form a key part of a semi dynamic datum for Nepal which could be based on be ITRF2014 with a reference epoch set some time after the end of the current sequence of earthquakes.

In order to test the effectiveness of a semi-dynamic datum to correct for the deformation from the April 25, 2015 Mw7.8 Gorkha earthquake, we adjusted GPS data that contained both pre and post-earthquake measurements. These test points define a polygon extending about 40 km in the NW SE direction centered on Kathmandu. Between these points there are nine GPS baselines, three of which were recorded in April 2013, before the earthquake and six of which were observed on 08 May 2015, in the period between the 25th April 2015 Gorkha Earthquake and the 12th May Mw7.3 aftershock. The first adjustment was conducted without using a deformation model while, in the second adjustment, the deformation model was used to correct all the measurements to pre-earthquake values. The Standard error of unit weight for the adjustment which does not apply the NDM is more than a factor of 3 times greater than the SEUW for the model which does apply the NDM. This difference demonstrates that the deformation model is effective in correcting for crustal deformation between the two surveys.

Control

It would be desirable if top level control for Nepal semi-datum was be based on a CORS network with coordinates being rigorously aligned to the ITRF. In Nepal, this network could make use of stations from the Nepal GPS Array (a network established by Caltech and run by UNAVCO to study earth deformation). A preliminary evaluation of the stations in the array indicates that 20 of these sites may be available to act as a CORS network for Nepal. However, four sites download only sporadically due to problematic data links, which results in long latency periods. The distribution of potential CORS stations is shown in Figure 5.

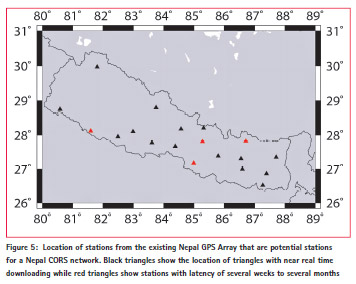
Coordinates for existing lower order coordinates would be determined by readjusting existing measurements combined with new surveying data, particularly in the Kathmandu area. Least square adjustments must use packages that can apply the deformation model correctly. This will require that observation dates are available for all historic measurements and it will require that the data is available in a digital form stored in a suitable geodatabase. Comparing the old and new coordinates from existing control will allow a series of correction grids to be developed and these will provide the basis of an accurate datum transformation between the modernized and original Nepal datums. The correction grid will be used to transform coordinates and spatial references in geodatabases into the new system.

Tools for surveyors to connect to the new datum

Nepal will need a mechanism to allow surveyors to realize coordinates in the new datum. This can be done by establishing a network of control coordinates. In Nepal, topographic maps are published in sheets covering 7.5 arcminutes of latitude and longitude in the densely populated regions. The less populated high mountain regions are mapped on 15 arcminute sheets. If high level control points were established at a density of one per topographic base map sheet, this would give a density of about 1 station per 10-15 km in the populated regions of Nepal and one per 25-30 km for the mountainous areas. High level passive control marks, which have been used in previous earth deformation studies, should be resurveyed as a matter of urgency as they can contribute to the velocity model. For points that will contribute to the velocity field, processing must be done using techniques that provide coordinates and velocities that are aligned rigorously to the ITRF such as Bernese GAMIT or GIPSY.. For new static control points which are not intended to be used for measuring velocities, processing using commercial software will be adequate. Once the new stations have been established and surveyed, the data will be adjusted relative to the CORS to determine coordinates in the new datum. This requires the use of adjustment software that supports the use of deformation models, for example SNAP from Land Information New Zealand (LINZ 2013) or HTDP (Pearson and Snay 2012). Sufficient points with existing Nepal Everest coordinates should be included to allow the transformation grid to be developed.

In addition to the network of passive control marks, Nepal could consider developing web based products and other infrastructure to allow surveyors to connect their surveys directly to the CORS network. At its simplest this might take the form of hosting RINEX files from the CORS on its own website, or even training Nepali surveyors to use the existing UNAVCO web interface for this purpose. However, the UNAVCO web interface has some limitations for providing data in the form that surveyors can use easily. For this reason, it would be desirable to have RINEX data served locally in Nepal on a server under the control of the Survey Department.

Eventually, it may be possible to incorporate, at least some of the CORS network in a NetworkRTK facility. In this regard, some of the stations in the Nepal GPS Array are already producing high rate GPS data so incorporating them in a Nepali NetworkRTK should not be particularly difficult although the reliability of communication links and data latency may be a problem. It may also be possible to develop capacity for online data processing along the lines of the existing PositioNZ-PP, AUSPOS or OPUS services.

Conclusions

Because of the effect of the 25th April, 2015 Gorkha earthquake, significant earth deformation has occurred in a large area of Nepal centered on the Kathmandu Valley. As a result, the geodetic control in this region is significantly distorted with published geodetic control coordinates being displaced from their true position on the ground by up to 2m. Correcting these distortions will require a new geodetic datum. In this paper we consider the possibility of Nepal adopting a semidynamic datum, which would be based on ITRF2014 and include a national deformation model capable of correcting for the recent earthquakes and normal tectonic motion. We demonstrate that it is possible to develop a deformation model for Nepal incorporating the Gorkha earthquake and the variation of the long term (or secular) crustal velocity across the country using published information. While this model is preliminary our test shows that its use does a good job of correcting survey measurements for the effect of the earthquake.

References: and Further Readings

Galetzka, J. et al., (2015). Slip pulse and resonance of Kathmandu basin during the 2015 Mw 7.8 Gorkha earthquake, Nepal imaged with geodesy, Science, 349(6252): 1091- 1095. doi: 10.1126/science.aac6383.

National Geodetic Office LINZ (2013) SNAP Guidelines for GNSS Geodetic Control – CSV files SNAP version 2.3.60, Wellington New Zealand http://www.linz.govt.nz/system/ files_force/media/file-attachments/ Guidelines-SNAP-v1.0_0.pdf

Pearson, C., Manandhar, N., and Denys, C., (2016). Towards a modernized geodetic datum for Nepal: Options for developing an accurate terrestrial reference frame following the April 25, 2015 Mw7.8 Gorkha earthquake, Online Proceedings of the FIG Working Week 2016, Christchurch New Zealand, in press.

Pearson C and Snay R. (2012) Introducing version 3.1 of the Horizontal Time-Dependent Positioning utility for transforming coordinates across time and between spatial reference frames, DOI 10.1007/s10291-012- 0255-y GPS Solutions doi:10.1061/ (ASCE)SU.1943-5428.0000013

Spence F S. (1987) Himalayan Survey, The Geographical Journal, Vol. 153, No. 2, pp. 223-230

Stanaway, R., Roberts, C., Blick, G., and Crook, C., (2012). Four Dimensional Deformation Modelling, the link between International, Regional and Local Reference Frames, Online Proceedings of the FIG Working Week 2012, Rome, Italy, 6-10 May 2012